Wind Load on a Shop – Envelope Procedure – Part 3 – Vertical (Uplift) Forces

In an earlier example (here) we looked at the total horizontal force acting on an 8-foot `swath’ of a shop building, the idea being that the shop is supported by trusses on posts at 8 foot (ft) on center (o.c.), and that the horizontal wind force would be shared by any cantilever action of the columns, and the roof acting as a horizontal diaphragm. The assumption was that the force would include only the top halves of the windward and leeward walls; the wind forces on the lower halves of the walls carried either by the lower portions of the columns, or through some kinds of wall bottom plate,, down into the foundation. We found that the total horizontal force, for 8 feet of wall, to tops of posts, was 500 pounds (lb). The solution was a bit interesting, as it was derived, per the requirement of Section 28.3.3 (ASCE 7-22) that we ignore the effect of the wind of the roof. (More about that in a different post.)

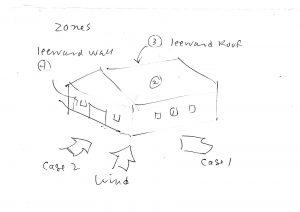
Now let’s look at the total horizontal and vertical wind forces, going clear down to, but excluding, the foundation. The total horizontal force will be easy, since we will again end up neglecting the roof; we end up using the force(s) on the whole walls, 1,000 lb (see the previous post).

Now let’s get the vertical force(s).

Both the windward and leeward roof planes span 20 ft, and rise 4/12 or 6.67 ft. The slope length, bottom to top, of each, is √(20² + 6.67²) = 21.1 ft. Both roof surfaces, then, for an 8-ft swath, have areas of 8 ft x 21.1 ft = 169 square feet (sf). Again we’ll omit the eaves, addressing them in another post, or posts.

In the earlier example we considered the shop `partially enclosed’, allowing for a large door open at one end, and the other end closed. The corresponding internal pressure coefficient, GCpi, is +/- 0.55. Since we’re looking at vertical forces, particularly `uplift’, we’ll use the +0.55; the internal pressure is pushing upward on the underside of the roof, inflating the building. This internal pressure will `add’ to the suction of the external pressures, helping it `explode’.

From the previous example the external pressure coefficients are GCpf (windward roof, Zone 2) = -0.69 and GCpf (leeward roof, Zone 3) = -0.47.

The Velocity pressure and Directionality factor, from that example, are, respectively, qz = 18.5 psf, and Kd = 0.85.

The pressures on the roof surfaces, p = qz Kd [(GCpf) – (GCpi)], are:

p (windward slope) = 18.5 psf (0.85) (-0.69 – 0.55) = 18.5 (psf 0.85) (-1.24) = -19.5 psf, and

p (leeward slope) = 18.5 (0.85) (-0.47 – 0.55) = 18.5 psf (0.85) (-1.02) = – 16.0 psf.

The resulting forces (F = p x A; 8-ft swath) are, thus,

F (windward slope) = 19.5 psf x 169 sf = 3296 lb, say 3300 lb, and I leave off the minus sign knowing it’s suction (outward and upward), and

F (leeward slope) = 16.0 psf x 169 sf = 2704 lb, say 2700 lb, also outward and upward.

The actual vertical components of these forces are,

Fy (windward slope) = 3300 lb cos (18.4°) = 3130 lb; and

Fy (leeward slope) = 2700 cos (18.4°) = 2570 lb.

The total vertical forces is … 3130 lb + 2570 lb = 5300 lb.

Whoa! The vertical (uplift) force is over five times that of the horizontal force. All other things equal, this building will have a greater tendency to lift off the ground and `fly away’, versus just being pushed along the ground from the horizontal components of the wind action. The shop acts more like an `airfoil’ than a `blunt’ obstacle to the wind.

*****

The walls, per these calculations, do not contribute directly to the (purely) vertical wind load, but we can talk more about that, later.

TAGS: wind, force, load, wind load, ASCE 7-22, Envelope Procedure, horizontal, vertical, gust, coefficient, internal, external, GCpf, GCpi, wall, roof, zone, enclosed, partially enclosed