Wind Load on a Shop Using ASCE 7-22 (Envelope Procedure) (Part 1 – Velocity Pressure)
Part 1 – Velocity Pressure
Let’s consider a shop to be constructed in, say, Latah County, Idaho, near the recently publicized college town of Moscow. The shop will not be a `business’, per se; in other words, people will not be there non-stop during `business hours’; it is a shop for a farm. Oh, it will, at times, quite possibly, house farm animals. The dimensions are 40 feet (ft) by 100 feet. The walls are 8.5 ft tall (on 6-inch stemwalls); the roof is supported by trusses @ 8 ft on center, and the roof slope is 4/12. Yes, the roof has eaves on the sides and rakes at the ends, which we will ignore for now. (Note that I go back and forth between present and future tense, as this shop-barn largely already exists, but in another location.) The roof trusses are pre-manufactured metal-plate-connected, and will be supported at the side walls by, 6 x 6 wood posts.
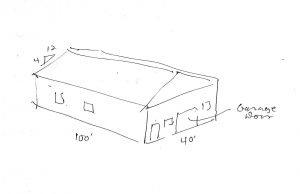
Of interest, in this post, is the sideways (lateral, or horizontal) loading on the upper portion of the walls, and roof, due to a `big wind’. It is this `big wind’ that we need to `design against’, so that the shop doesn’t flop over. We will assume (for now) that the framing of the shop (roof membrane, roof purlins, trusses, wall plates, and wall girts), collect the wind load so as to deliver to deliver it to the tops of the wood support posts. (Maybe I should call the posts `columns’, as this whole blurb is a blog `post.)
I recently received hard copy of the 2022 Edition of the American Society of Civil Engineers Minimum Design Loads and Associated Criteria for Buildings and Other Structures (ASCE 7-22), Provisions and Commentary, and am anxious to dig in! Chapters 26 through 30 (of ASCE 7-22) address Wind Loads; we will look specifically at Chapters 26 and 28; Chapter 26 gets us started with General Requirements; per our shop being a low-rise building, and, Figure 26.1-1, we are invited to use Chapter 28 to get the wind load on the `Main Wind Force Resisting System’ (MWFRS), using the Envelope Procedure. In this `post’, with respect to the MWFRS, we will focus on a single `frame’ (non-moment) or `bent’ consisting of a truss and the posts at ends, collecting wind load from an 8-ft wide `swath’ of windward wall, roof, and leeward wall (4 ft each side, or to the next trusses and posts). Table 28.2-1 gives us the Steps to Determine Loads on MWFRS Low-Rise Buildings.
Here goes.
Step 1: Determine the Risk Category … Table 1.5-1. Okay; Risk Category I (per the intended use described above).
Step 2: Determine the Basic Wind Speed, V, per Risk Category, from Figure 26.5-1. In our case it will be Fig. 26.5-1A; I get 96.5 miles per hour (mph) (rough interpolation).
Step 3: Determine the wind load parameters:
→ Wind directionality factor, Kd, Chapter 26; we get … Kd = 0.85.
→ Exposure Category, Section 26.7 … it’s `fairly open’ … `C’.
→ Topographic Factor, Kzt … the western portion of Latah County is in the `Palouse’, a region of rolling hills, previously covered in grass and perhaps Camas, now largely agricultural land planted in wheat, lentils, garbanzo beans, and other crops. `Our’ structure will be in a relatively flat area (not on a hill, or hilltop); we’ll use Kzt = 1.0. (There are many places in the Palouse where Kzt = 1.0 would not be so, and some that very much have a topographic effect; ask me how I know.)
→ Ground Elevation Factor … cool … this is relatively new in `the code’ … the provision to take into effect the different density of air at different altitudes, or elevations. (Less-dense air generates less wind force as it slams into things, like buildings.) I will use an elevation of 2500 ft; interpolating from the values in Table 26.9-1, I get Ke = 0.915. (Note that I show this to 3 significant figures, which is probably pure fantasy, in terms of the air slamming into my building … but that’s the way we do engineering … often portraying far greater precision than is, in most cases, ever justified.)
→ Enclosure Classification … I did the actual calculations offline; I get `Partially Enclosed’. I got the classification based on there being large garage-type doors at the ends, one of which might be open, and the other closed, during the design wind event.
→ Internal Pressure Coefficient, GCpi, Section 26.13 … GCpi = +/- 0.55. Now, what is interesting is this: because our bents are symmetric side-to-side, the internal pressures on the walls cancel out; “0.55” will not show up in the calcs (calculations) on this `post’, since I’m only looking at the horizontal (lateral) wind loading. Likewise the horizontal effects of the internal pressures on the roof cancel out. BUT, the internal pressure coefficient will VERY MUCH show up when we look at uplift (vertical) forces on the roof.
Step 4: Determine the velocity pressure exposure coefficient, Kz or Kh, from Table 26.10-1 … I get 0.85, using Exposure Category `C’, and a mean roof height of 15 ft (or less). (The mean roof height is … 8.5 feet + ½ of ½ of 20 ft x 4 / 12 = 8.5 ft + ½ of 6.7 ft = 11.8 ft. Actually, there will be at least a 6-inch tall stem wall, above ground, so 11.8 + 0.5 = 12.3 ft … still 15 ft or less … Kz = 0.85. (I wish this were a different number than that for Kd, so as to keep the two better sorted out; oh well.)
Step 5: Determine the velocity pressure, qz or qh, from Equation 26.10-1 …
qz = 0.00256 Kz Kzt Ke V2 … in the English System (for which I make no apology) …
qz = 0.00256 (0.85) (1.0) (0.915) (96.5)2 = 18.5 psf …
(Since the V is in units of mph, the 0.00256 must carry with it the units of pounds per square feet (psf) pressure, per mph `squared’ … it is essentially comes from the units-independent energy equation, divided by volume … ½ ρV2.)
I like to call this the `stagnation’ pressure’, or the `stagnation pressure @ 33 ft, Exposure C’ … the pressure the air molecules deliver to the surface of an object upon slamming into it and coming to rest … transferring their kinetic energy into increase pressure.
(If you don’t think this pressure is real, try carrying a 4 ft x 8 ft piece of plywood, held upright, across the street on even a moderately windy day.)
(We’ll deal with wind loading on signs in a different post.)
This is getting kinda long … we’ll look at pressure coefficients, pressures, and (horizontal) forces, in … Part 2.